MORPHOLOGY STUDIOS 2013-2014
We continue explorations of minimal surfaces and their tie-in with various constructive and fabrication technologies to understand how complex 3-dimensional forms can be modeled and built using digital and analog methods.
Ajmal Aqtash
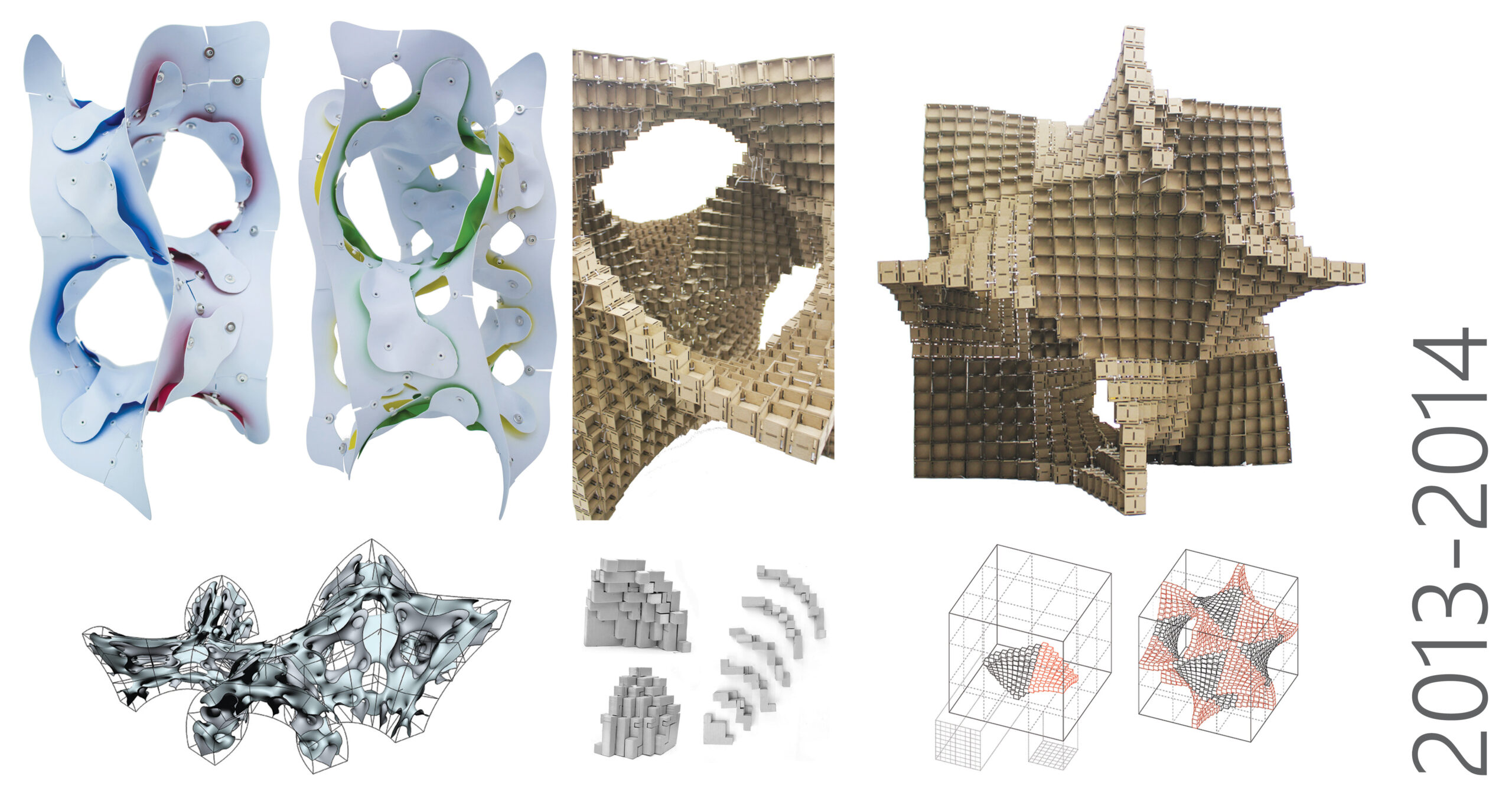
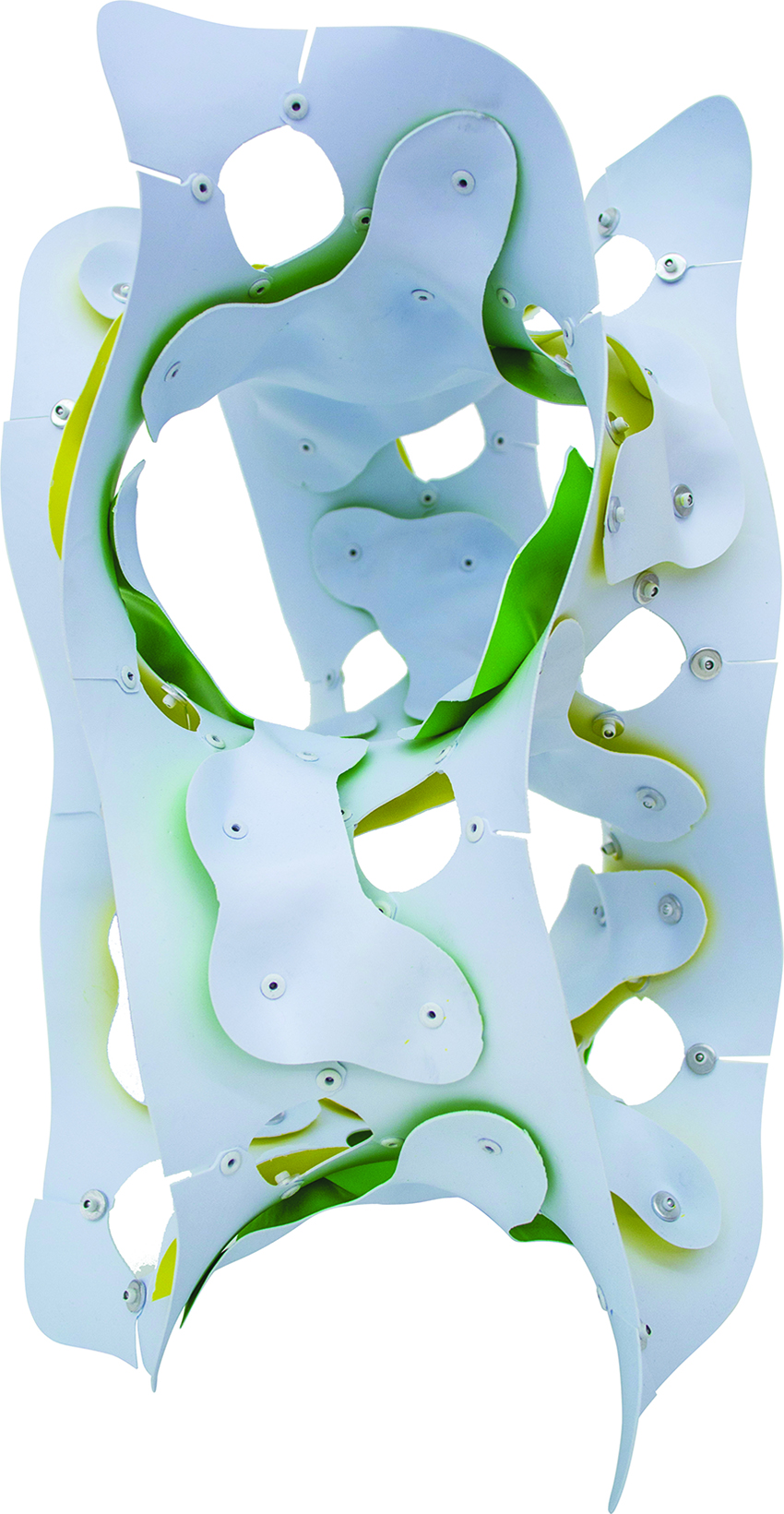
This is a multi-semester project with the aim of building a complex surface (Schoen’s gyroid) from modules that are increasingly scaled up in size using the same material (styrene) and same method of forming. Individual saddle-shaped modules, vacuum-formed and CNC trimmed, are joined by metal rivets and grouped together for an indoor installation. Double-layered modules having dimpled surfaces enable stronger surfaces from the same thickness of material and allow larger spans. We are currently scaling up the module for a larger outdoor installation involving robotic fabrication.
Students: Brandon Conde, Catherine Lim, Charlene Liang, Georgia Reyes, Jasper Hayes, Jay Liu, Jef Offenbecher, Yoskiki Mino, Alexander Smith, Alex Vasilyev.

Patrick Donbeck/Haresh Lalvani
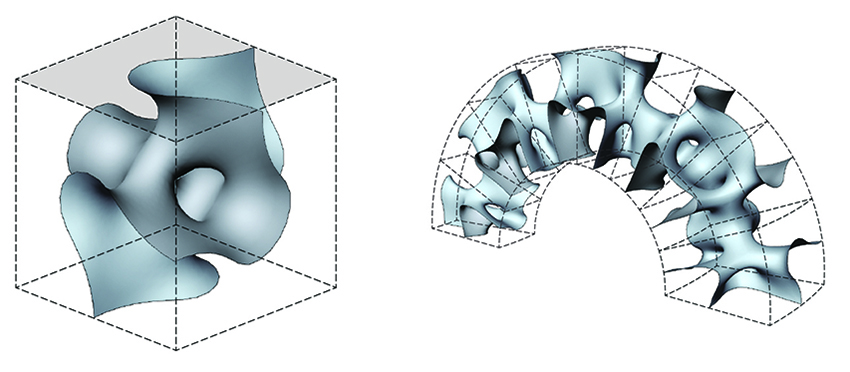
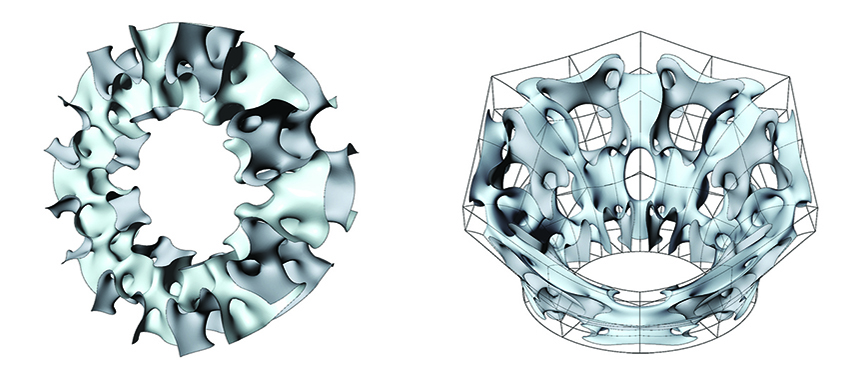
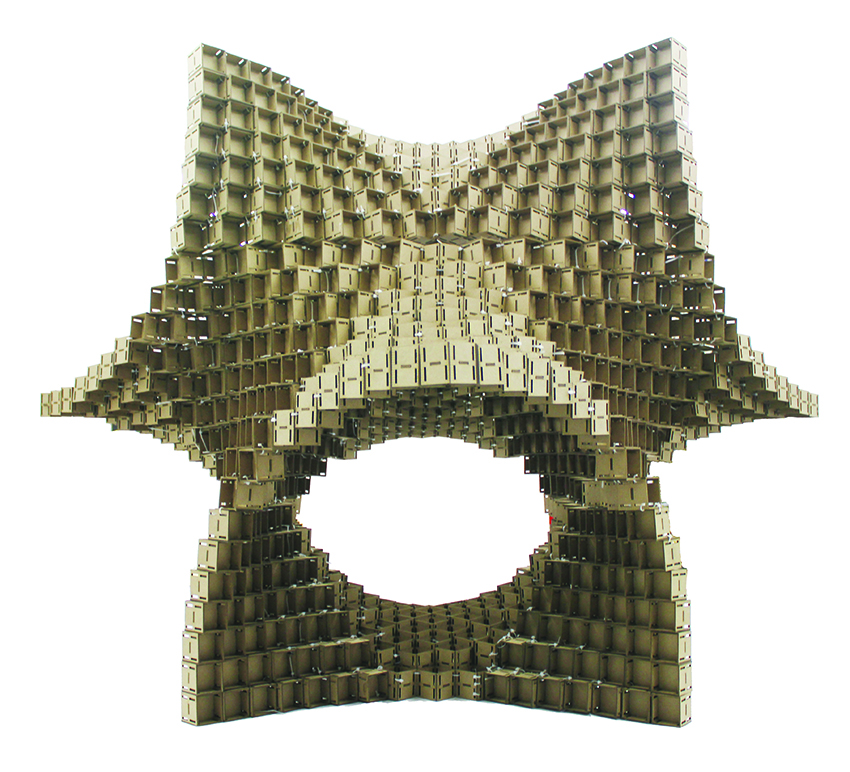
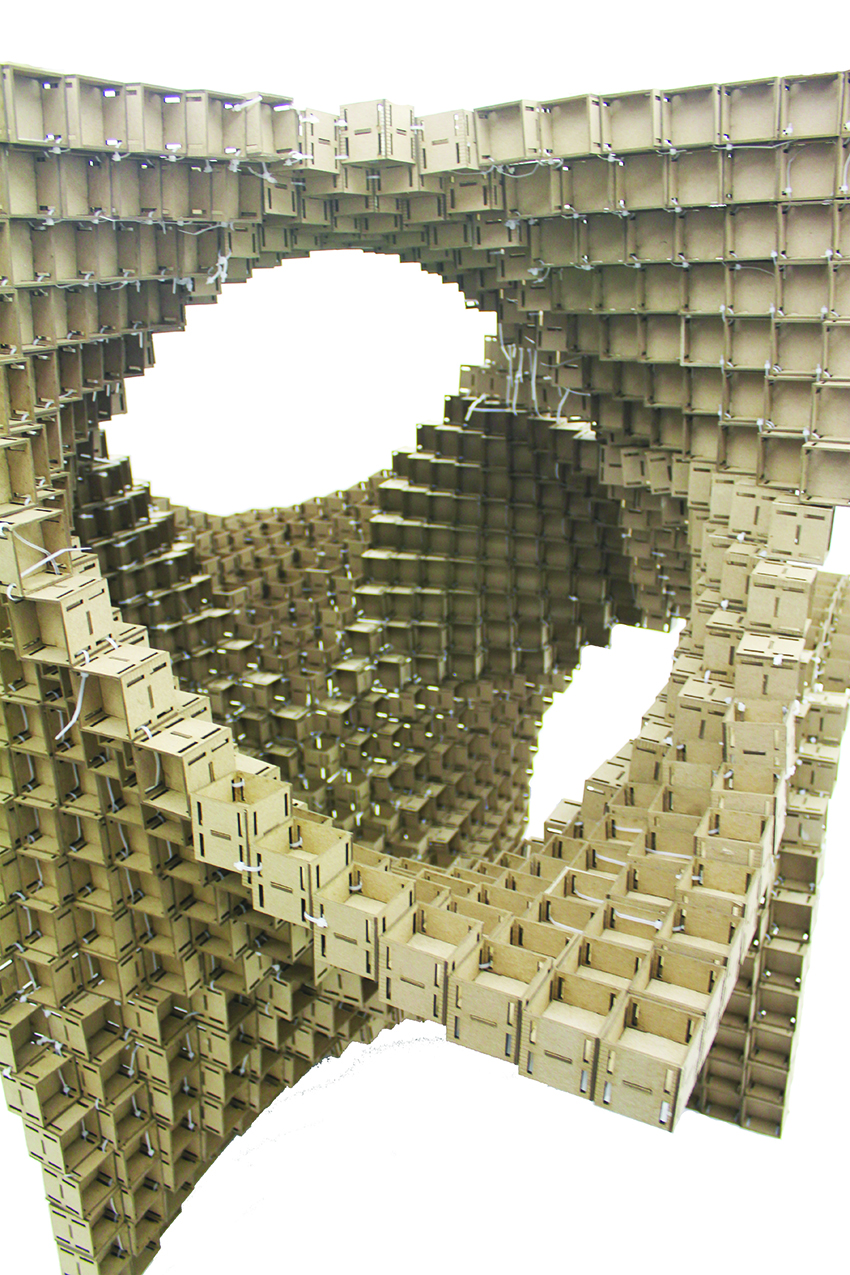
This group of studies focused on using a single 3D pixel (voxel) as a constructive element for fabricating curved surfaces with positive and negative curvatures. Inspired by the great vaulted and masonry/brick work of Rafael Guastavino and Antonio Gaudi, we explored the formal possibilities of a universal voxel unit, a cube in this instance, to systematically construct complex surfaces. This culminated in a group project to build a large voxelated minimal surface shown here and based on a curved variant of a well-known “infinite polyhedron”, a class of continuous space-filling surfaces that divide space into two distinct parts, inside and outside, in the same way that a simple box does with the topological difference that the box is “closed” while our surface is “open”.
Students: Hilary Flannery, Nicole Mattos, Hayden Minick, Georgia Reyes, Robinson Strong, Ali Tiner.

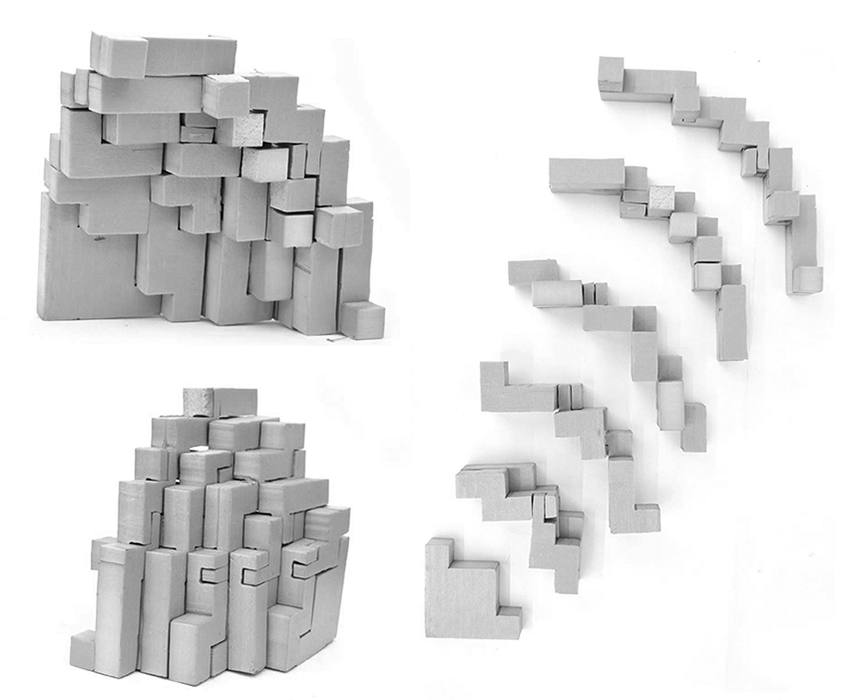
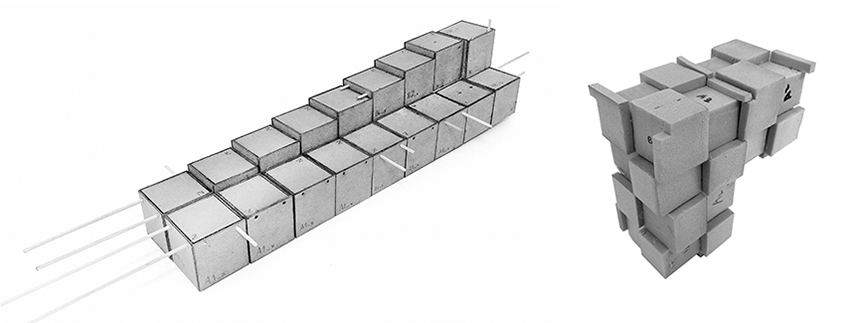
John Gulliford/Haresh Lalvani
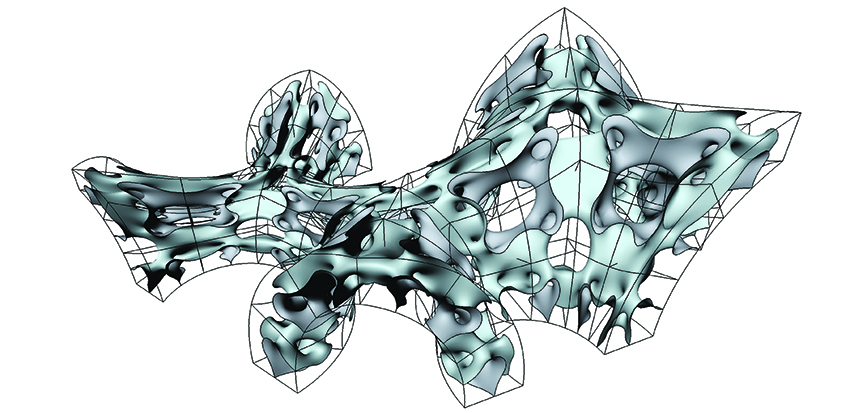
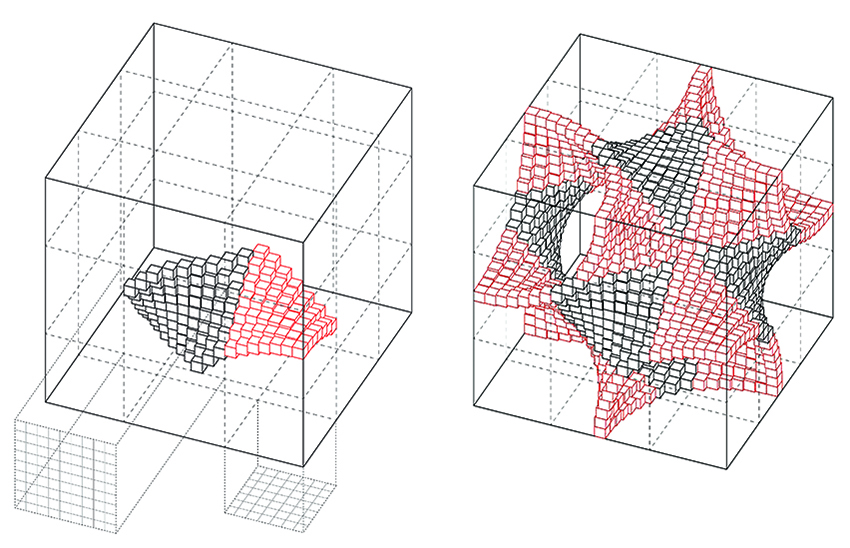
Various morphing techniques are used to generate a complex surface starting from a single cell through continual step-by-step transformations that lead from symmetrical to asymmetrical spaces. The cell of a non-Euclidean minimal surface is first derived using several fundamental regions, the minimum modules need to construct the surface. We use Brakken’s taxonomy which describes the shape of this fundamental region as a “Manta Ray”. This cell is repeated to produce a complex surface with a high genus (number of holes). The surface morphs gradually as the underlying space morphs from Euclidean to non-Euclidean curved space. Students explored architectonic possibilities based on these digital studies.
Student: Georgia Reyes